Hybrids at hand: the problem of representing the heterotic superstring
Contrasting conventional notions of representational realism with the leaps of imagination underlying contemporary physics, Sean Miller explores the necessary role of an imaginary in sting theorists' search for a coherent "theory of everything."
This paper was presented originally at the 2008 MLA Convention in San Francisco. It was part of a panel entitled “Beyond Representation: New Work in Literature and Science,” chaired by Professor Henry Turner of Rutgers University and sponsored by the Division on Science and Literature.
In this essay I will analyze a prominent technical article from string theory in order to offer up an alternative to a “realist” notion of representation, one that is often imposed problematically on theoretical physics. Published in the journal Nuclear Physics B in 1985, the article “Heterotic String Theory” is the result of a collaboration between four physicists from Princeton University, David Gross, Jeffrey Harvey, Emil Martinex, and Ryan Rohm. I chose this article for the reason that a particular version of it is considered by many string theorists to be the most realistic version of string theory to date. Because it is considered realistic - in a specific sense that I will elaborate on shortly - an examination of the exposition that supplements the mathematical formulation of the heterotic string within the technical article lends itself to the question of the adequacy or inadequacy of such a representational realism.
In Women, Fire, and Dangerous Things, Lakoff paraphrases this conventional notion of representation in a way that I think is readily applicable to theoretical physics in its attempt to formulate a realistic theory such as the heterotic string: According to conventional notions of scientific realism, a physical theory should be: (1) The manipulation of abstract symbols that are meaningless in themselves and get their meaning only by virtue of correspondences to things in the world. Accordingly, (2) theory is abstract and disembodied, a matter of instantiating some transcendental rationality. And (3) Theory is thus a “mirror of nature,” and as such, it constitutes an “internal representation of an external reality.”
Before tackling the expository content of the article itself and how a certain reading of it suggests that the realism that I just described is inadequate to encompass its textual complexities, I will summarize briefly string theory’s most salient features. String theory is a physical theory that attempts to reconcile quantum theory with general relativity. Quantum theory explains the workings of the microcosm in terms of the interaction of subatomic point-particles that, depending on their attributes, are defined as either matter - also known as fermions - or three fundamental forces, electromagnetism and the strong and weak nuclear forces, often referred to as bosons. General relativity describes the workings of the macrocosm - entities on large scales, such as planets, stars, galaxies, and the universe as a whole. It does this by delineating a relationship between matter, space, time, and a fourth fundamental force, gravity. Limited to their respective domains, both theories are highly predictive - quantum theory at the smallest of scales where gravity has negligible effect and general relativity at the largest of scales where the force of gravity dominates. But in scenarios where the scales meet, for example, black holes or the early universe just after the Big Bang, both theories break down and produce nonsensical predictions. They become mutually incompatible. String theorists seek to reconcile quantum theory with general relativity by theorizing one mathematically consistent formalism that incorporates both quantum theory and gravity - a quantum theory of gravity. They do this by arguing that the most fundamental constituent of the universe is not a point-particle, but a string, a tiny one-dimensional extended object that vibrates. The frequency of the vibration of the string determines the kind of quantum particle it manifests. Matter and force quantum point-particles, including the graviton, are different excitation modes of one fundamental string.
In order to accommodate many of the prerequisites for a physical theory to be realistic, the heterotic string model, in particular, takes on numerous counterintuitive characteristics. The most noteworthy example of this is the fact that in order for the most realistic versions of string theory to be mathematically consistent, they call for not the four dimensions of spacetime - three for space and one for time - in quantum theory and general relativity, but rather twenty-six or, in some cases, ten dimensions of spacetime. The problem of imagining this multitude of spacetime dimensions becomes further complicated in the case of the heterotic string.
A technical article such as “Heterotic String Theory” generally consists of two basic components that, together, form a coherent narrative whole: there is mathematical argument and surrounding that mathematical argument, expository prose. According to a realist conception of physical theory, the mathematical argument comprises the conceptual content. It possesses whatever truth-value the article may have. The exposition that surrounds the mathematical argument is a supplement - it is meant to simply help orient the reader and to serve as a form of pedagogic “illustration” of the truth-content. Such an understanding of a technical article as text sorts mathematic argument as concept and the surrounding exposition as image, with a subordinate status. A relation of abstract symbols, rigorously delineated, corresponds to things in the world. That correspondence is substantiated through experiment. Experimental validation of theory lends legitimacy to that theory’s status as an accurate internal representation of external reality, or, in other words, as a “mirror of nature.”
The issue of how and why a “thing” in the world comes to gain a phenomenological legitimacy is more properly the purview of sociologists of science. A constructivist account of the phenomenological substantiation of an entity such as the heterotic string would put a premium on the historically and culturally situated embodiment of physicists as they conduct experiments to validate theory. But what interests me in the reading of an article such as “Heterotic String Theory” is not so much the sociology of physicists, whether theorists in offices or experimentalists in laboratories, but rather, the necessary role of an imaginary in this regime. It is to look at yet another missing ingredient in this epistemological system that includes an external reality - a “world” - and a disciplined method for physical intervention into that world, which is a complex aggregate of mathematical argument and experiment. The missing ingredient, generally unacknowledged, is this imaginary that resides in the exposition that surrounds mathematic argument in the technical article. The philosopher Michéle Le Doeuff argues that “imagery and knowledge form […] a common system […in which] there is a play of feedbacks that maintains the particular regime of the discursive formation” (5). For Le Doeuff, such images in the text allow it to reintroduce into the discourse that which the conceptual content cannot resolve in and of itself and subsequently hopes, in some sense, to evade. The imaginary in the expository content affords a means, as Le Doeuff puts it, “through which subjectivity can be structured and given a marking which is that of the corporate body” (5).
At the beginning of the article, the authors provide a summary description of the “heterotic” string:
[T]he heterotic string is constructed as a combination of the right-moving coordinates of the ten-dimensional superstring and the left-moving coordinates of the 26-dimensional bosonic string. This yields a theory which is Lorentz invariant in D = 10 and which has appealing features not found in either theory separately. […] Thus the name ‘heterotic.’ (254-5)
A theory that is “Lorentz invariant” is one that is consistent with special relativity, a pre-condition for any realistic physical theory. This particular version of the string is “super-” because the model incorporates supersymmetry, a theoretical structure that, in pairing both fermions and bosons with their hypothetical superpartners, allows for the synthesis of the standard model’s three distinct forces into one “supergroup.” For mathematical consistency, the superstring must reside in a spacetime of ten dimensions: the “D” in the passage signifies “dimension.” The position of the heterotic string is “embedded in ten-dimensional flat space-time” (256).
Gross et al. choose the qualifier heterotic (from “heterosis”), exploiting the word’s sense of “different” or “mixture,” since their new model joins ten-dimensional fermionic superstring theory with 26-dimensional bosonic string theory. The idea here is that while the entire theory is embedded in ten dimensions of spacetime, there is also another level, so to speak, of structure. This is the theory’s “attribute” space, an abstract space that bears with it the attributes of the strings being treated, in particular, their mass, winding number, and “left-” or “right-moving” spin. It is this attribute space, with its own dimensionality, that is “embedded” within spacetime. In the heterotic superstring imaginary, we find one abstract space, attribute space, coupled to and interacting with another abstract space, spacetime.
They go on to describe yet another feature of the heterotic string:
The new feature is the treatment of the 16 internal, left-moving, bosonic coordinates. We show that these must, for consistency, be compactified on a particular torus. This compactification leads to a set of 496 massless vector bosons. (255)
Dividing the 26 left-moving bosonic coordinates into two groups, they designate 16 of these as “internal,” implying that the remaining 10 are thus external. Here then we have the image of a space within a space within a space. An “internal” container-like space of 16 left-moving bosonic coordinates is embedded within a container-like attribute space that couples yet leaves distinct the remaining 10 left-moving bosonic coordinates and the 10 right-moving fermionic coordinates. This imagined space, in turn, is embedded within a ten-dimensional “flat space-time” abstract space. Since the external space of left-moving bosonic coordinates is 10-dimensional, each coordinate within that space contains a supplementary container-like “compact” 16-dimensional space. Gross et al. describe this supplementary space as having “hidden, compact spatial dimensions” (253) and are concerned with “the structure of the internal sixteen-dimensional space” (258). That structure must, “for consistency, be compactified on a particular torus.” A torus is a donut-like geometric object defined mathematically by the notion that some spatial dimensions are flat while others are positively curved. So the imaginary, in addition to placing a space within a space within a space, is further complicated by a multiple multi-dimensionality that is not only imagined as flat space but also as curved space, a “compactified” torus of 16 dimensions.
My point here is not to call into question the science, but rather to highlight the extraordinary nature of the imaginary that accompanies what are considered consistent mathematical arguments. The imaginative achievement is in the retrofitting of basic human-scale images - string, dimension, space, and implicitly, basic spatio-temporal kinaesthetic relations such as inside and outside, left-moving and right-moving, action-as-event, linking, container - to a imaginative domain where there can be no expectation that such phenomena as they are generally known and recognized on human scales would at all be pertinent or viable. The impression of utter weirdness of such imaginaries is very much a product of their heterogeneity, the medley of abstract space within space, object within object, where no schema in particular would seem to stand out as the one monologically coherent worldview.
Significantly, an image of reality-as-hidden-depth envelopes the argument. It reinforces the substantiation of string-as-depth, as fundamental substance that lies within or below the form of the real world, and out of which quantum particles - whether bosons or fermions - manifest. An embodied praxis via experiment ultimately validates mathematical arguments as precise propositional constraints on an imagined relationship with the natural world. As such, a kind of folk epistemology implicit in the exposition surrounding the mathematical argument grants it an imaginative substance and affective resonance, tapping, as it does, the conventionality and cultural currency of an imaginative regime of reality-as-depth. In our knowledge, we may mark the heterotic string as the deeper truth.
In their concluding remarks, the authors concede:
It is certainly true that the formulation of the heterotic string appears somewhat awkward and contrived. This is not a shortcoming of our theory; rather it is indicative of the present level of understanding of all quantum string theories, which leaves much to be desired. Many of the most remarkable features of these theories emerge without a full comprehension of their origin. […] This suggests that there exists a more profound formulation of string theory, in which these features would be manifest. In such a formulation the heterotic theory might appear more natural. (282)
I find this defence of the theorists’ version of string theory remarkable for a number of reasons. Implicit in the admission that their formulation “appears somewhat awkward and contrived” is a conflation of imaginary with conceptualization (through mathematics). If a given mathematical argument is self-consistent and correct, how can it be awkward and contrived? This admission further makes use of, once again, the epistemic binary of appearance and reality, where appearance is “awkward and contrived,” whereas, by implication, reality would be the opposite, that is, elegant and coherent. But is there any a priori reason why reality - as it is imagined - decidedly should not be awkward or contrived? The theorists then blame this “shortcoming” of the theory not on their own formulation, but on the “present level of understanding of all quantum field theories.” Again, a future “level of understanding” would presumably go “deeper.” Immanent in their defence is the imaginary that physical reality - and our capacity to understand it - consists of “levels” and that a “more profound formulation” would somehow capture a deeper reality, and accordingly, “might appear more natural.”
What we must contend with is that as mathematical arguments within string theory technical discourse become ever more complex, the imaginary that accompanies theory becomes all the more counterintuitive and bizarre. This is not merely the case of what Michel Serres calls the “local monstrously inflated,” but rather, a multitude of heterogeneous locals recombined and projected into various remote scales (Genesis 3). To contend with these remote scales, the imagination must either radically shrink its own proxy agency to a scale appropriate to the microcosm, or conversely, magnify the microcosm in order to enable human-scale imaginative access.
I offer the following analogy to better imagine the process by which theorists manage the three elemental components of their theorizing, which I have referred to throughout as mathematics, imaginaries, and embodied praxis (experiments), in order to produce string theory - a kind of epistemic triangulation. All are indispensable to scientific practice and together they are mutually constituting. Mathematics provides precise prompts for action - constraints that guide but do not represent. As an essential complement, the imaginary affords a means of sharing among practitioners a taken-as-cohesive experience of remote spaces and alien encounters - the substantive content to mathematical form. An imaginary mediates between body and world and grounds mathematics to a community with its own particular social conventions, that is, to a culture. This culture structures both affect and relationship - what to feel about the abstract spaces, how to feel it, and how to behave toward the phenomena that populate those spaces.
Therefore, one can imagine heterotic superstring theory - its mathematical arguments, abstract spaces, and abstracted human and natural agents - functioning in a way analogous to the epistemic regime of a high-powered microscope. A theorist “sees” a virtual medium constructed through a series of feedback loops from the intervention of abstracted hands with putative “objects” in a remote space. In the analogy, the microscope is akin to prosthesis: it allows access by a human body to remote spaces on extreme scales. Mathematics, in turn, allows for the precision necessary to make the instrument sufficiently sensitive to interact effectively with these remote objects, ultimately allowing for the proper discernment of their behavior.
This relationship between mathematics, imaginaries, and praxis helps to explain why the further one “delves” into “nature,” the more counterintuitive and just plain weird it seems. There is absolutely no reason that objects and events that have gained their phenomenological stability from “common-sense” experience - from human bodies and minds interacting with the world on human scales, where this experience is further conditioned by historical and cultural contingencies - ought to obtain on scales far removed from those scales. According to Lakoff and Turner, in terms of the scientific knowledge evinced by string theory technical discourse, a relevant question is: “How can we manipulate our conceptual resources in such a way that we can create ways of understanding other things in terms of ourselves?” (74). I have argued throughout that such a redeployment of “conceptual resources” allows string theorists to understand the “things” about which they are concerned not simply in terms of “ourselves,” but more specifically, in terms of the full cache of human-scale objects, events, and spaces, however abstracted from their origins, available to our imaginations. It is a testament to the facility of the imagination that string theorists are able to re-combine, re-scale, and re-deploy images that originate at or near this “home” to far-flung spaces and make them at all capable of facilitating repeatable, effective physical intervention into those realms.
It would seem that grappling with any given representation of the heterotic superstring may teach us this: we can only expect that the further we push back the “boundary” of the known into the unknown, the imaginaries through which we mediate that encounter with “natural origins” will become ever more hotchpotch, ever more, as Serres puts, “a mixture, tiger-striped, motley, mottled, zebra-streaked, variegated” (Genesis 111). Though this radical heterogeneity is not uniformly stochastic: patterns, logics, and regularities may organize local structures. There is, nonetheless, no ironclad expectation that declares that a given imaginary that affords us access to remote spaces should in any way be coherent or unitary. In the case of string theorists, they are at complete liberty to blend images in any way they see fit. Ironically, it is perhaps the theorists’ longing for a coherent “theory of everything,” a “master equation,” as Brian Greene puts it, that provides the impetus to cobble together an ever more disparate imaginative heterosis - with all its hybrid vigor (Elegant 5). While the heterotic string, on first glance, simplifies the disparate quantum particles of the standard model into one core image, namely, the string, that image itself has no relevance outside of a complicated bricolage of yet more images. A push to elegance and cohesion would only seem to displace elsewhere the counterintuitive complexity requisite for ostensibly representational exposition to match the arabesque intricacies of mathematical argument.
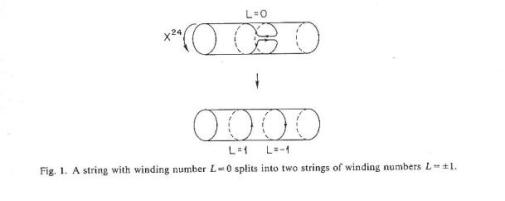
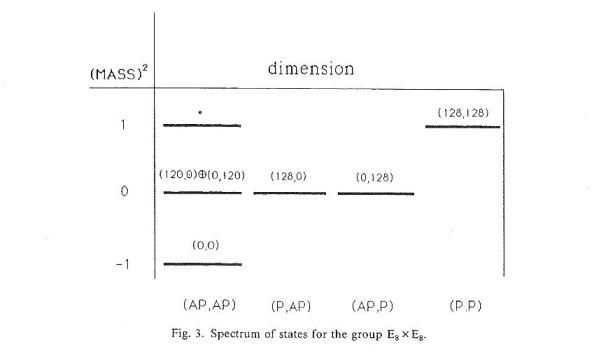
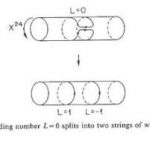
Here are two examples of illustrations from the article. They constitute representational fragments of the radically heterogeneous string theory imaginary - a knee or tusk-view, so to speak, of the whole elephant, of which no coherent illustration is available.
Works Cited
Green, Michael B., John H. Schwarz, Edward Witten. Superstring Theory, 2 vol. Cambridge: Cambridge U. Press, 1987.
Greene, Brian. The Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory. New York: Random House, 1999.
Gross, D.J., J.A. Harvey, E. Martinec, and R. Rohm. “Heterotic String Theory (I): The Free Heterotic String.” Nuclear Physics B256 (1985): 253-84.
Lakoff, George. Women, Fire, and Dangerous Things: What Categories Reveal About the Mind. Chicago: U of Chicago Press, 1987.
Lakoff, George and Mark Turner. More Than Cool Reason: A Field Guide to Poetic Metaphor. Chicago: U. of Chicago Press, 1989.
Le Doeuff, Michèle. The Philosophical Imaginary. Trans. Colin Gordon. London: Continuum, 2002.
Serres, Michel. Genesis. Trans. Genevieve James and James Nielson. East Lansing, MI: U. of Michigan Press, 1997.
Zwiebach, Barton. A First Course in String Theory. Cambridge: Cambridge U. Press, 2004.
Cite this essay
Miller, Sean. "Hybrids at hand: the problem of representing the heterotic superstring" Electronic Book Review, 28 April 2009, https://electronicbookreview.com/publications/hybrids-at-hand-the-problem-of-representing-the-heterotic-superstring/